Music Modes: Major and Minor Modal Scales in Music Theory

This post about music modes was excerpted from lesson 7 of Paul Schmeling’s Berklee Online class, Music Theory 201.

The Construction of Major Music Modes

The term modal scales is applied to a group of scales commonly used in pop and jazz music. Music modes are different than the “regular” major and minor scales most students are familiar with. Each mode has a name, and mode names come from the Greek language and from a time before major and minor (as we know them) were clearly defined.

The scale we now know as major was originally called the Ionian mode and its relative minor was known as Aeolian.

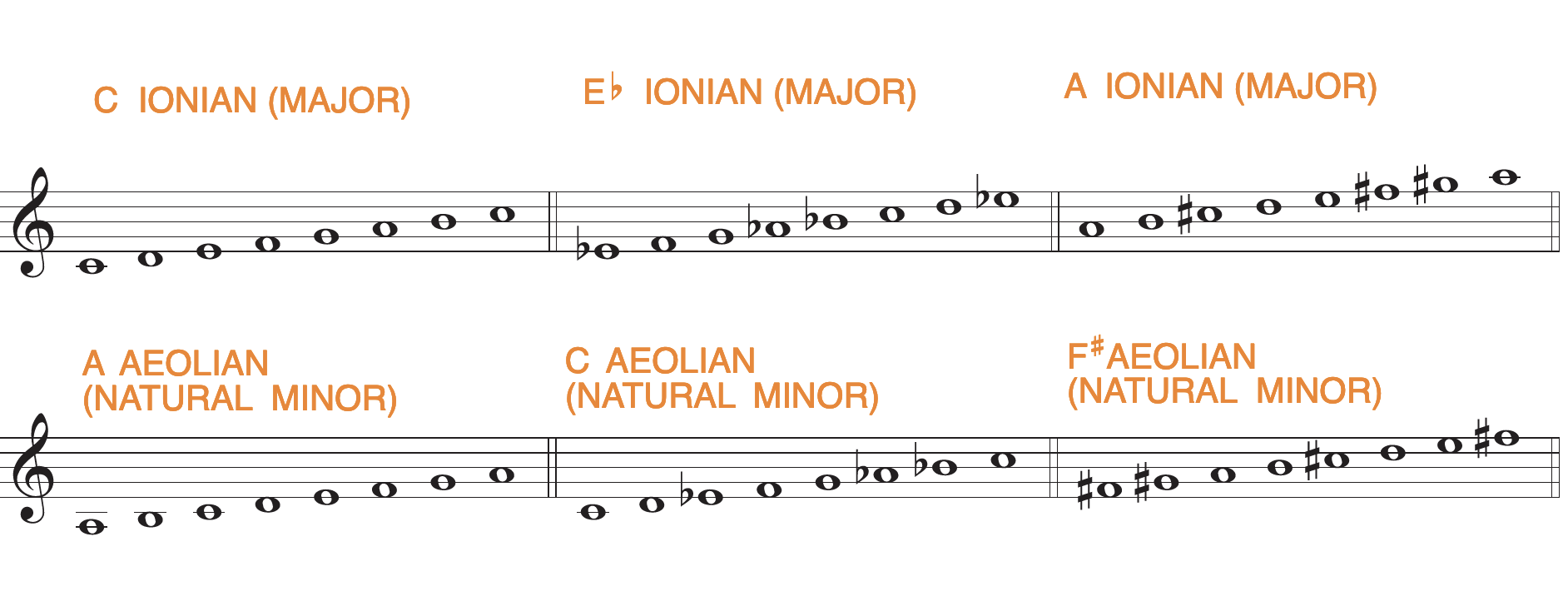
We will use these two commonly known scales as a point of reference, as we look at the modal scales.

Let’s look at the three major music modes first: the Ionian, Lydian, and Mixolydian, each of which has major 3rds.

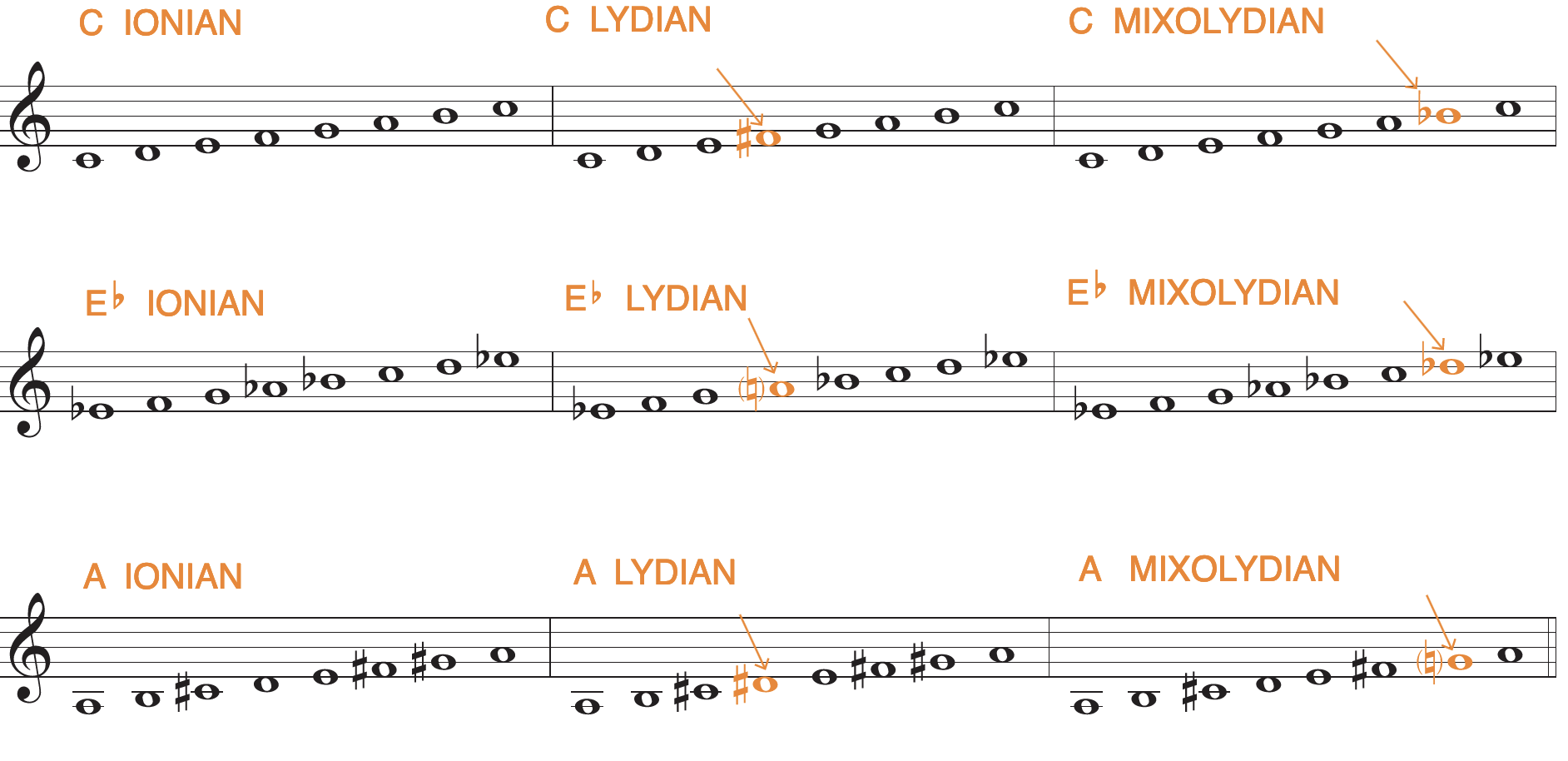
Each mode has characteristic notes—particular notes that clearly set each apart from the regular major, or Ionian, scale. For example, notice that the 4th degree of the Lydian scale is a half step higher than its counterpart in the Ionian or major scale, and the 7th degree of the Mixolydian scale is a half step lower.

When we talk about what key a piece of music is in, we often describe both the tonality (the tonic pitch), and the modality (the type of scale on that pitch). For example, “A minor” tells us the tonic pitch is “A” and the type of scale is “minor.” Using the same terminology, if we say a piece of music is in “G Mixolydian,” we are saying that the tonic pitch is “G” and the type of scale based on G is “Mixolydian.”

Here is a simple melody in the key of C major. Listen to how its character changes as it is “adjusted” to become first a Mixolydian, then a Lydian melody.

There are four minor modes (those with minor 3rds), and we have already seen one of them, Aeolian. Since we are familiar with Aeolian—we know the scale by its other name, natural minor —we will use it as a point of reference. The following contrasts the Aeolian scale with the other three minor modes: Dorian, Phrygian, and Locrian.

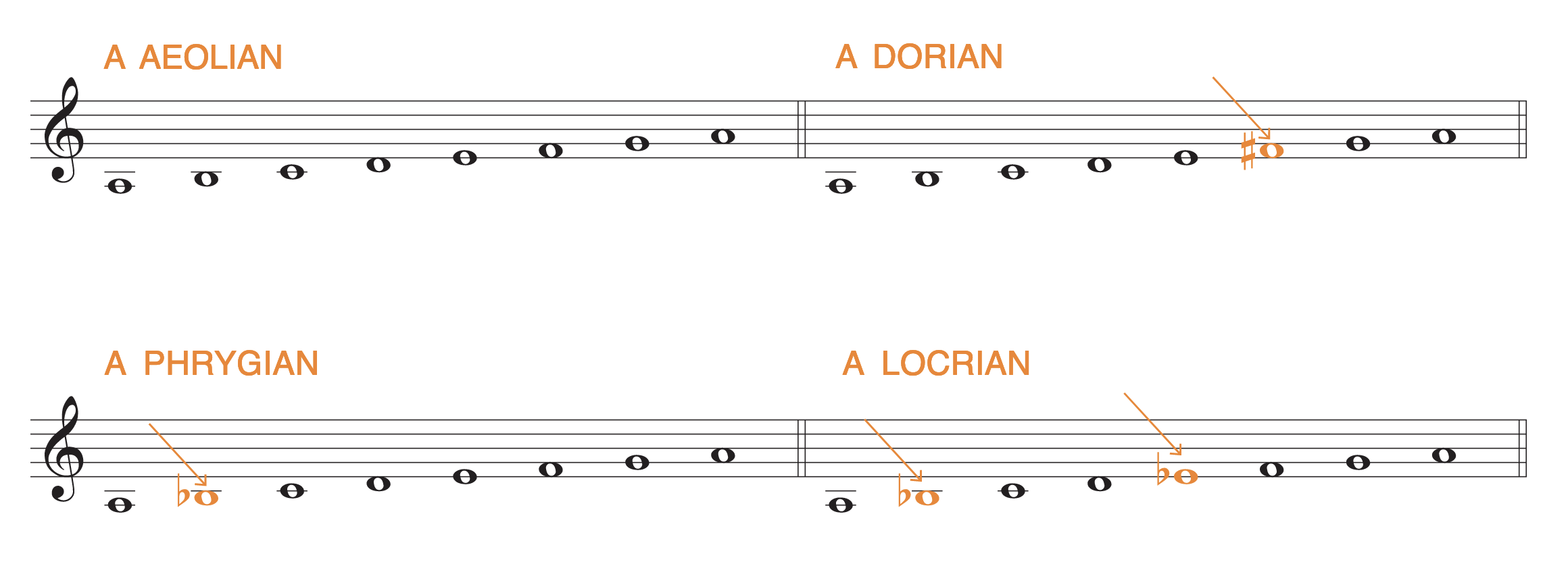
Notice that the Dorian scale has a 6th degree a half step higher than that found in Aeolian; the Phrygian scale has a 2nd degree a half step lower; and the Locrian scale has not only the lowered second degree but a lowered 5th degree, as well. These notes characterize the sound of these music modes, setting them apart from “regular minor.”

Let’s look at these minor modes on two other tonics—C and E.

Let’s revisit our melody and see how it sounds, first of all adjusted to Aeolian as written, then as Dorian, Phrygian, and Locrian.

Notice that Dorian sounds brighter than Aeolian, but Phrygian and especially Locrian have a much darker sound quality.

Writing Modal Melodies

Because our ears are more accustomed to hearing melodies in major, and to a lesser extent, “regular” minor, modal melodies have to work extra hard to promote their tonality and modality. Keep these points in mind as we approach our assignment, in which you will write a four-measure melody in each of the music modes:

1. Make use of all of the notes of the scale. In the following melody, scale degree 6 is not used, making it impossible to know if Dorian or Aeolian.

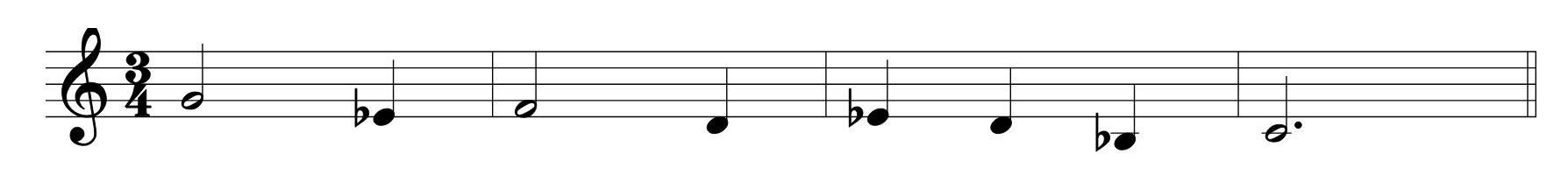
2. Make frequent use of the characteristic note of the scale. This melody could stand a few more F#s to ensure a Lydian sound.

3. Emphasize the tonic note by using it frequently and for notes of longer duration, especially those on strong beats. Assuming this melody is supposed to be in C Mixolydian, it needs more C notes—especially those of longer duration and falling on strong beats.

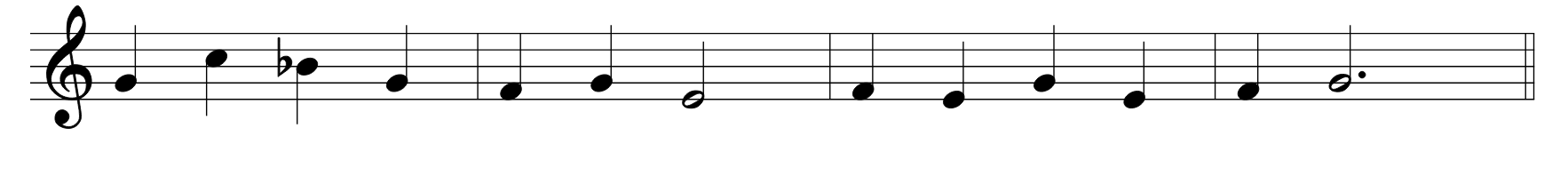
4. Use a “melodic cadence” of scale degrees 2 to 1 and/or 7 to 1 at the end of each of your four-measure melodies. Melodic cadences, like harmonic cadences, resolve more strongly toward the tonic pitch than other scale members, helping to give the tonic greater emphasis. Notice in the following example that the sense of rest (cadence) is much stronger on C, in the example below.

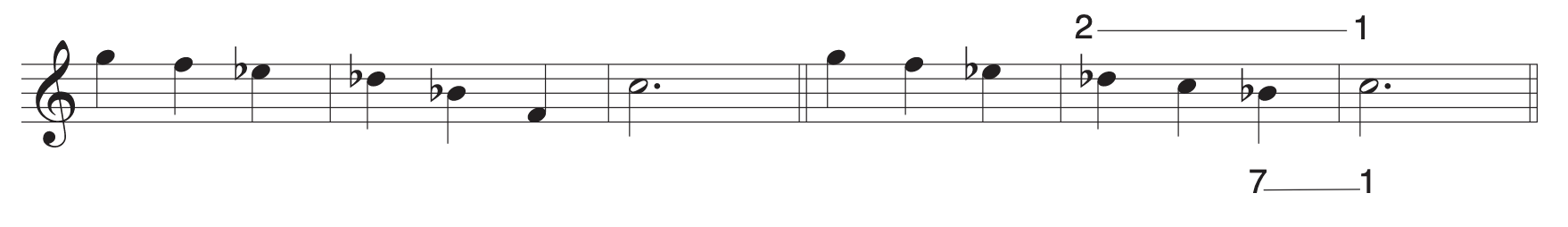
5. Avoid leaping or spanning the interval of a +4th or o5th, which occurs within each modal scale. This is an unstable interval with a strong tendency to resolve—but not to a place that we want it to go! It implies the dominant 7 of the relative major (the major scale with the same notes), and we do not want to go there. In the following C Dorian example, the interval E♭ to A, whether leaped or spanned, implies an F7, the dominant of B♭ major, and we do not want B as the tonic; we want C.

STUDY MUSIC THEORY WITH BERKLEE ONLINE